Earth, Wind, and Forces
The wind is moving air. The air begins to move when acted upon by unbalanced forces (see Vectors and Laws of Motion). Four fundamental forces affect air: pressure gradient force, friction, Coriolis force, and centrifugal force. Only one force does not depend on how the air is moving: pressure gradient force. The other three depend on how the wind flows.
Air Pressure
Air pressure is the weight of the column of air above that location and elevation. Pressure is force/area, and average sea level air pressure is 1.033 kg/cm2, 10,332 kg/m2, or 14.7 lb/in2. Another way to think about it: put a 15 lb bowling ball (or a jug of sand – see the experiment that follows) on every square inch of a surface exposed to air. The palm of a hand is on the order of 10 in2, so there are at least 147 lbs of air (10 bowling balls!) pushing against its surface. Luckily our blood, muscles, and bone push the skin back at the same pressure, creating balanced forces acting on our skin. If the forces weren’t balanced, it would get pretty messy.
Another standard unit for pressure is a millibar (mb). A bar is the pressure of one atmosphere, so a millibar is one-thousandth of a bar – or the weight of one-thousandth of an atmosphere. So 500 mb represents the weight of half an atmosphere.
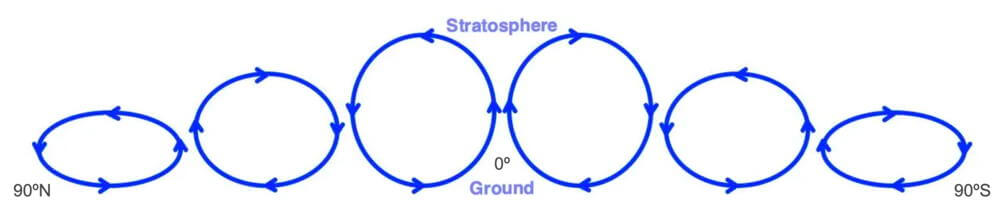
Idealized cross-section of the global circulation cells extending from one pole to the other (the North Pole is on the left and the South Pole on the right of the diagram). The cold air sinks at the poles and flows toward lower latitudes. Warm air rises near the Equator, which is consistently the warmest region.
The two tallest circulation cells that are within the tropics are called the Hadley cells. The midlatitude cells, or the two moderately tall cells, are called the Ferrel cells. The polar cells on the left and right of the diagram are the shallowest compared to the others.
See the Sun-Earth Connection to explore the Earth’s uneven heating by the Sun. The stratosphere is presented in detail in Atmospheric Layers.
Pressure Gradient Force
What gets air moving? It’s an unbalanced force resulting from uneven heating of the Earth’s surface by the Sun (see the Sun-Earth Connection) that creates a pressure gradient (change pressure over distance).
The air in the tropics warms by conduction at the surface and rises, and polar air cools by conduction and radiational cooling and sinks. This sets in motion three circulation cells (convection cells) between the tropics and each of the poles (see image above). The polar downwelling air is on both ends of the diagram, and the rising tropical air is in the middle of the picture.
Based on Earth’s rate of rotation, location of the continents and oceans, the presence of our stratosphere, and the temperature gradient between the Tropics and the poles, Earth has three large circulation cells per hemisphere. Why is there an odd number of circulation cells in each hemisphere? Why couldn’t there be two? If there were two circulation cells between the warm tropics and cold poles, the sinking air from the equatorial cell would run into the rising air from the polar cell, which would shut off the circulations. With this pattern in the global temperature gradient (cold at the poles and hot in the tropics), there must always be an odd number of cells in each hemisphere. The seasonal heating patterns cause the positions and heights of the circulation cells to change during the year.
How do we go from a temperature gradient to a pressure gradient? Air continues to rise as long as it is less dense than its surroundings. About 20 km off the ground in the tropics the air begins to warm. This is the beginning of the stratosphere, also referred to as the ozone layer (see Atmospheric Layers). The warm air in the stratosphere is less dense than the rising tropical air, so the air stops rising, and it spreads toward the poles. Since the effect of friction with the ground decreases with height, air moving near the stratosphere has negligible friction, so there is no resistance to flow. Air flows out of the top of the rising column of air faster than it can converge at the bottom of the column of air. There is less air in the column of air, which decreases air pressure at the ground, reinforcing the pressure gradient that creates convergence at the Equator.
At the poles, the sinking air near the ground slows by friction. Air flows into the top of the column of air faster than it leaves at the bottom, so surface air pressure increases. A pressure gradient has formed between the tropics and the poles, keeping these large circulation cells moving throughout the year.
Activity to Experience Pressure Gradient
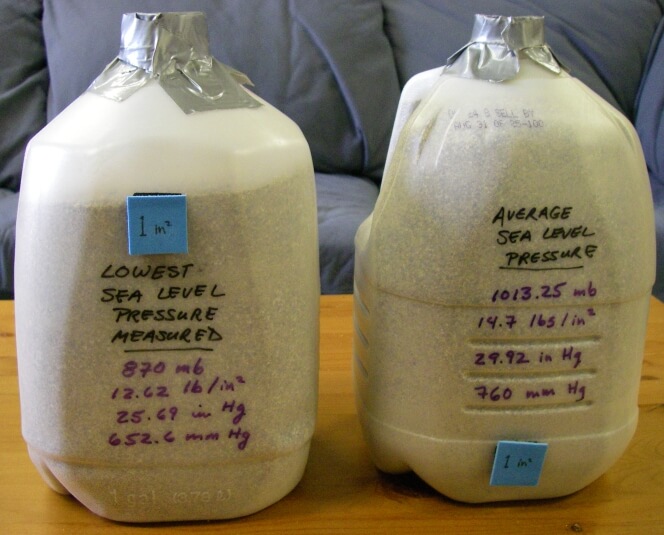
Put sand into two one-gallon milk jugs, so the first weighs 14.7 lbs and the second 12.62 lbs. The first represents the average sea level pressure, and the second is the lowest recorded surface air pressure.
Close your eyes and hold one jug in each hand. It is surprisingly difficult to tell which container is heavier. If there were the pressures separated by several hundred kilometers, this would have created one of the strongest hurricanes. It doesn’t take much of a pressure gradient based on our sense of touch to get air to move fast!
Tip: Cut 1-inch squares from durable material and velcro them to the jugs.

When sinking air reaches the ground, it must move away from the area to make room for more sinking air. The spreading out of air from a region is called divergence. Rising air creates a different pattern: convergence. Surface air must flow toward the area of rising air to maintain the rising column of air.
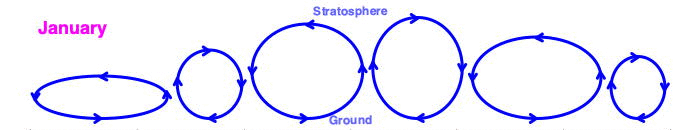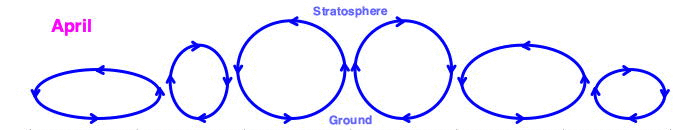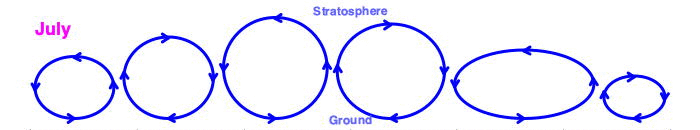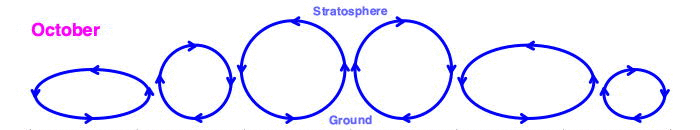
Above is an animation of the seasonal change of the global atmospheric circulation cells. The North Pole is on the left edge and the South Pole on the right side of the diagram.
When the two Hadley cells (the two tallest cells straddling the Equator) are similar in size, it is April or October or a month after the equinox. The major heating occurring around the globe determines the location of the upwelling air between the two Hadley cells, so it follows the seasonal heating patterns in the tropics. The warmest location should be the latitude where the Sun is directly overhead at local noon, but because the Earth’s materials take time to warm up, there is time delay as well as a spatial offset.
When the polar cell is at its tallest yet narrowest, it is the month after that hemisphere’s summer solstice. Since the poles remain the most consistently cold location in a given hemisphere, downwelling remains located at the poles throughout the year. Would this change is polar ice is lost?
See Sun-Earth Connection for more details.
Pressure Gradient and Isobars
Isobars are lines of constant air pressure. To learn how they are drawn from pressure measurements, see Contouring. That means air pressure changes most rapidly going perpendicular from the isobar. The pressure gradient is always perpendicular to isobars and points toward high pressure. See the three illustrations that follow.

The pressure gradient is the rate of change in air pressure over a horizontal distance. It is a vector and is drawn in red in the diagram above. Its magnitude increases as the isobars are closer together, and it points toward high pressure.
When the isobars are straight, it is easy to see that the pressure gradient is perpendicular to the isobars.
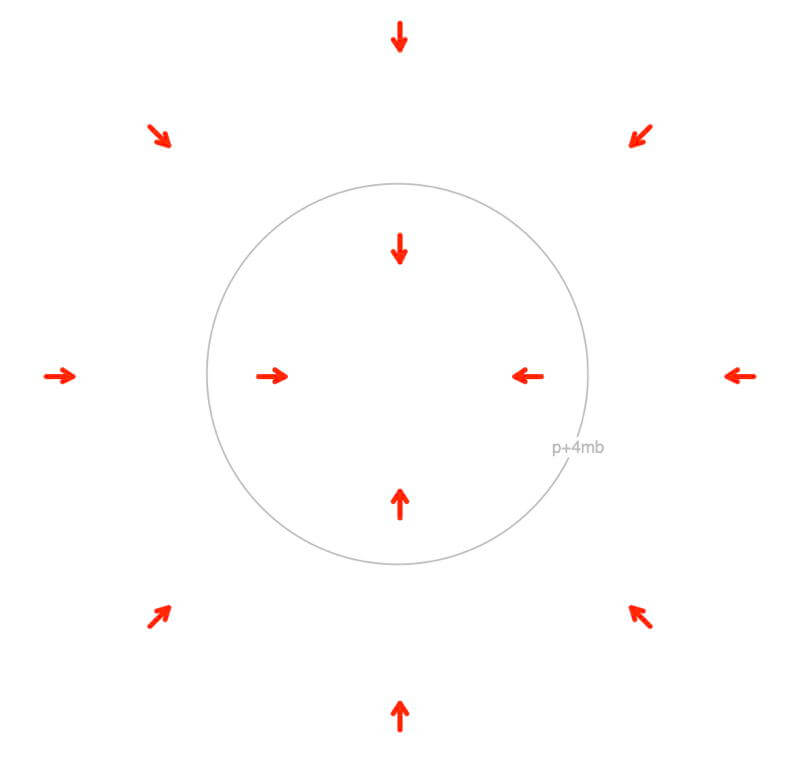
Isobars are curved for a low-pressure center, so the pressure gradient changes direction. Since the pressure is lowest in the center, the pressure gradients point toward the center.
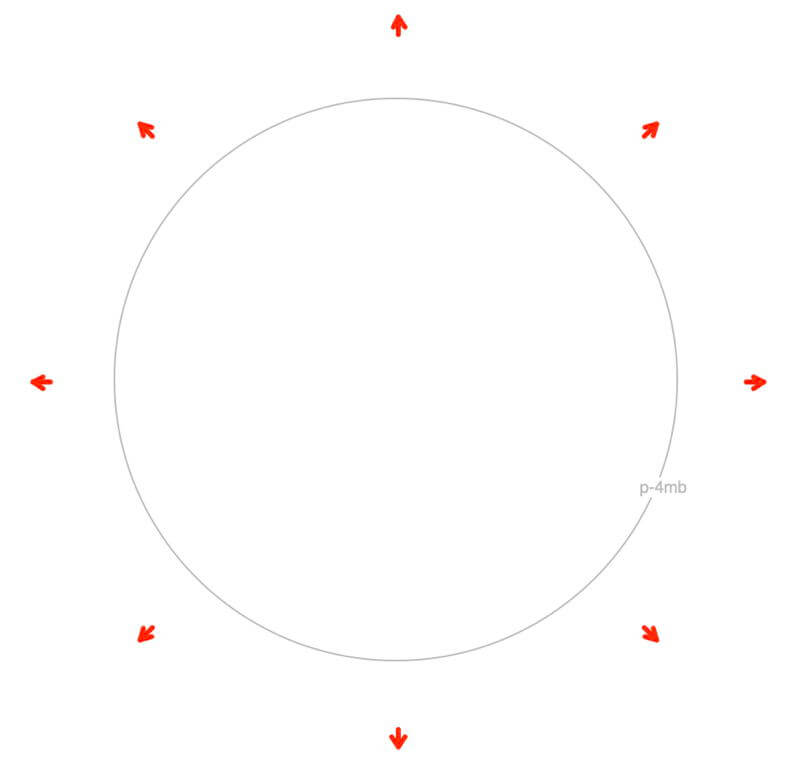
Isobars are also curved for a high-pressure center. Since the pressure is highest in the center, the pressure gradients point away from the center.
Friction
Friction is the force resisting the motion of objects, be it solid objects sliding past each other or moving fluid layers. There are a number of types of friction, but the one we will be dealing with for the fluids moving on Earth is fluid friction which describes the molecular interaction between layers of a viscous gas or liquid that are moving relative to each other.
Since friction opposes the motion of objects, it acts in the direction opposite to the motion and is proportional to the speed² of the moving fluid. When the fluid isn’t moving, there isn’t any friction acting on it. But when the fluid begins to move, friction increases quickly with the square of the speed of motion.

The friction with the grass opposes the motion of the air.

0 Comments